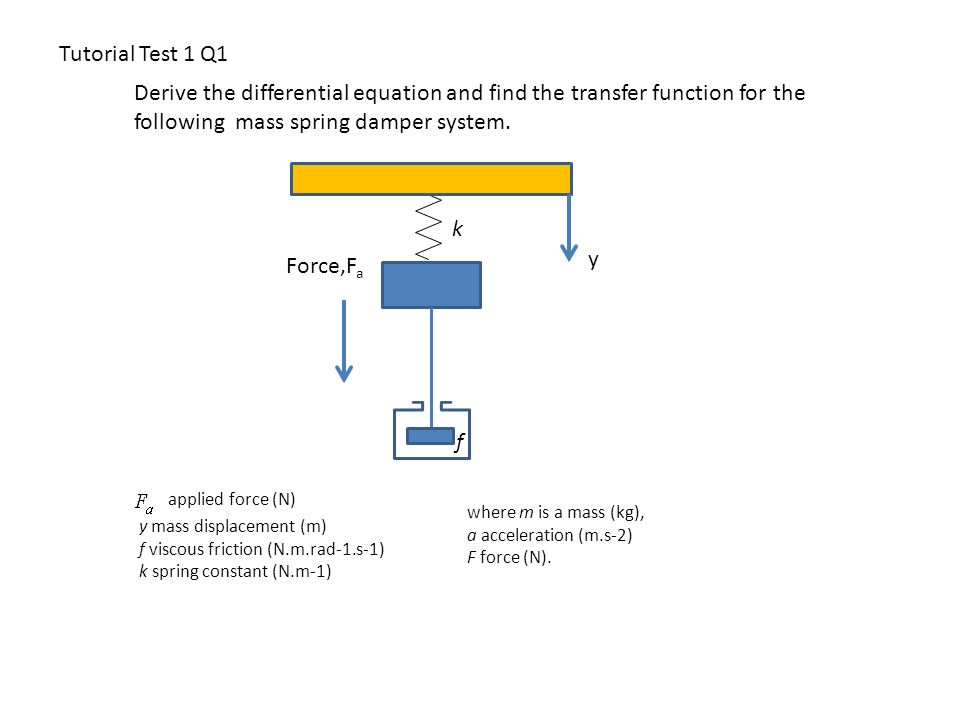
Spring Mass Damper System Differential Equation

This is a mass-spring-damper system equation with a function dependent on frequency M R K in parethesis. Based on Newtons second law. S2 4s 4 0. - F - 0150x 2 - 2x 1. Presents a canonical mass-spring-damper system and derives the governing differential equation. The damping coefficient c is simply defined as the damping force divided by shaft velocity. From the above equation it is clear that the period of oscillation is free from both gravitational acceleration and amplitude.

Legend Position Speed.

3 15 Transcribed image text. Legend Position Speed. Spring-mass-damper system If Fr and Fs are the only forces acting on the mass m then F ma where a dvdt x F m x Fs Fr -kx - bx So the differential equation for the mass position x is given by. Critical damping occurs when the coefficient of x is 2 n. The mass spring damper system is a physical system used to study mechanical vibrations. N 0 and call n the natural circular frequency of the system.

Differential Equation Modeling Spring And Mass Sharetechnote

Spring mass damper system differential equation. The general solution will be uleft t right u_cleft t right U_Pleft t right. When the viscous properties of the fluid in the buffer remain constant an ordinary differential equation of the second order serves as a mathematical model. Forward Euler approximation of the undamped mass-spring system h 001 The backward Euler method is defined as yn1 yn y n1h.

A-First order B-Second order C-Third order 2How can a dynamic system be put into motion. Dydt x 2. From the above equation it is clear that the period of oscillation is free from both gravitational acceleration and amplitude.

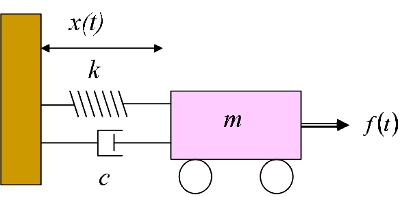
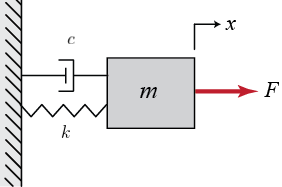
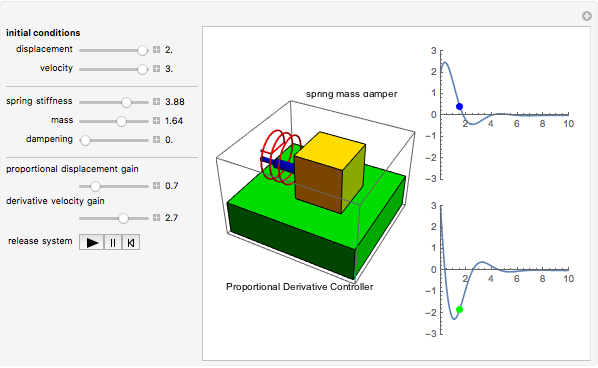
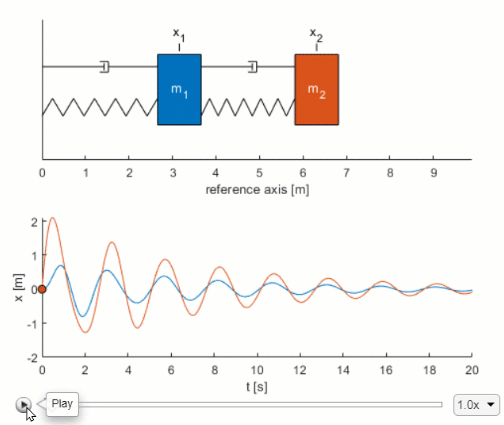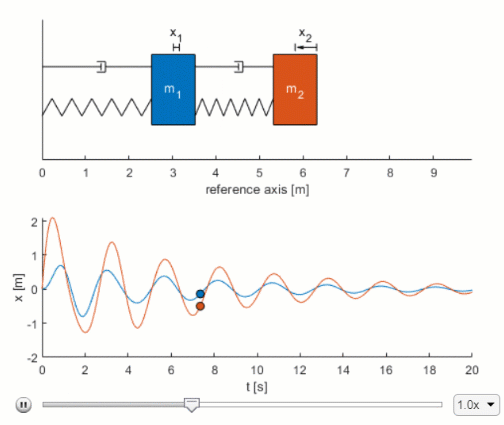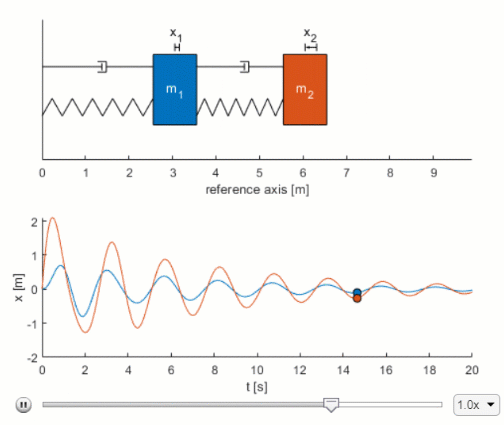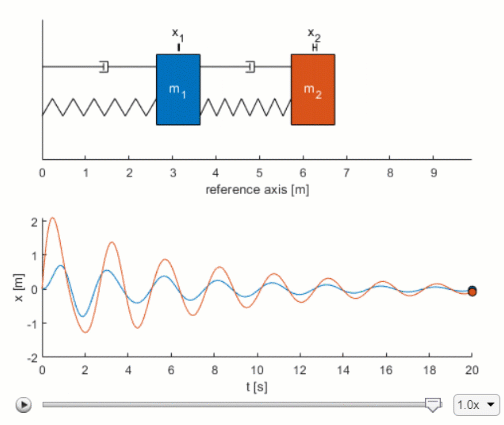
Presents a canonical mass-spring-damper system and derives the governing differential equation. Energy in a spring-mass system A horizontal spring-mass system has low friction spring stiffness 170 Nm and mass 0. The complementary function which arises solely due to the system itself and the particular integral which arises solely due to the applied forcing term.

S2 4s 4 0. Divide the equation through by m. N 0 and call n the natural circular frequency of the system.

Because the roots are repeated the system is critically damped. So x t e 2t 1 2t. That is to say the evolution of said mechanical oscillations can be predicted when studying the analytical solution of said equation.

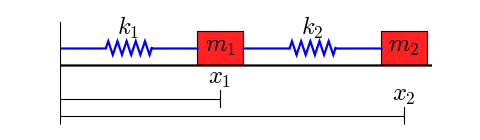
So we have a mass spring system which is supposed to be modelling a bridge and the equation that the displacement of the bridge x satisfies is given by. Ylabel Position Speed m ms xlabel Time s title mass-spring-damper system. 0 1 2 3 4 5 00 02 04 06 08.

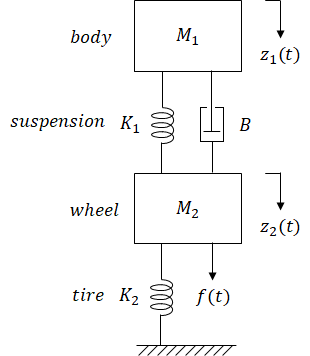
This factors 2 2. BACKGROUND MATH The time domain equation of motion for the mass-spring-damper is represented by Newtons Second Law written as the fol-lowing force balance on a structure 𝐌 𝐱 𝐂 𝐱 𝐊 𝐱 M Mass C Damping K Stiffness.

Mass Spring Damper System Download Scientific Diagram

Dsc Mass Spring Damper Concept Youtube

Solved 4 The Two Mass Spring Damper System Below Can Be Chegg Com

Modeling A Mass Spring Damper System Physics Forums

Solved Consider The Mass Spring Damper System Of The Figure Chegg Com

Enotes Mechanical Engineering

Differential Equation Modeling Spring And Mass Sharetechnote

Class 02 Ode Modeling Spring Mass Damper Dynamics Youtube

1 Program In C Or Python To Solve The Differential Equation Using Heun S Method For Mass Spring Damper System 2 Plot Vs And Vs On Course Hero

Ebook Engineering Analysis Using Scilab And C

Solved Consider The Mass Spring Damper System Of The Figure Chegg Com

Mec3403

Mass Spring Damper Tutorial Youtube

Tuned Mass Spring Damper Design Application Center

302 Vibration Of Mass Spring Damper System 2 Memorandums Of A Novice Automotive Engineer

Mass Spring Damper System

Single Degree Of Freedom Mass Spring Damper System Download Scientific Diagram

Differential Equation Modeling Spring And Mass Sharetechnote

Question8 N The Spring Mass Damper System In Figure 8 The Force F Is Applied To The Mass And It Homeworklib

Free Vibrations Of A Spring Mass Damper System Wolfram Demonstrations Project

Programming Exercises Mass Spring Damper Simulation Md At Master Enesdemirag Programming Exercises Github

Consider The Following Spring Mass Damper Mechanical System The Input Is Given By F T And The Output Is Y T A Use Free Body Diagram To Conduct Force Analysis B Use Newton S Law Of Motion

The Laplace Transform Of Modeling Of A Spring Mass Damper System

1

Spring Damper Calculation Plotting Stack Overflow

Mass Spring Damper System Tutorial Test 1 Derive The Differential Equation And Find The Transfer Function For The Following Mass Spring Damper System Ppt Download

Restackor Spring Mass Damper Theory

Solved Determine The Solution Of The Following Differential Chegg Com

Engineer On A Disk

Numerical Solutions Of Differential Equations Chms Tutorials 0 1 Documentation

Modelling Forced Oscillations With Second Order Ode S Steemit

9 Spring Mass Damper Systems Practical Matlab Modeling With Simulink Programming And Simulating Ordinary And Partial Differential Equations Dev Guis

Coupled Spring Mass System Scipy Cookbook Documentation

1 9 The Mass Damper Spring System A 2nd Order Lti System And Ode Engineering Libretexts

Spring Mass Damper System 1dof Youtube

Car Suspension System

Differential Equation Modeling Spring And Mass Sharetechnote

Techteach Simview

Robust Tuning Of Mass Spring Damper System Matlab Simulink

302 Vibration Of Mass Spring Damper System 2 Memorandums Of A Novice Automotive Engineer

1 There Is A Mass Spring Damper System As Shown In Fig 1 A Find The Total Response X T In Homeworklib

Obtaining Steady State Solution For Spring Mass Dashpot System Stack Overflow

16 311 Homework 3

Solved Solving Differential Eq Using Simulink Wheelchair

State Space Representation Of A Mass Spring Damper System The Robotics Lab

Spring Mass Damper Smd System With Proportional Derivative Pd Controller Wolfram Demonstrations Project

Github Mathworks Teaching Resources Mass Spring Damper Systems Interactive Courseware Module That Addresses The Fundamentals Of Mass Spring Damper Systems Taught In Mechanical Engineering Courses

Mass Spring Damper System Dynamics Dademuchconnection

1

Xbmx n2x 0.

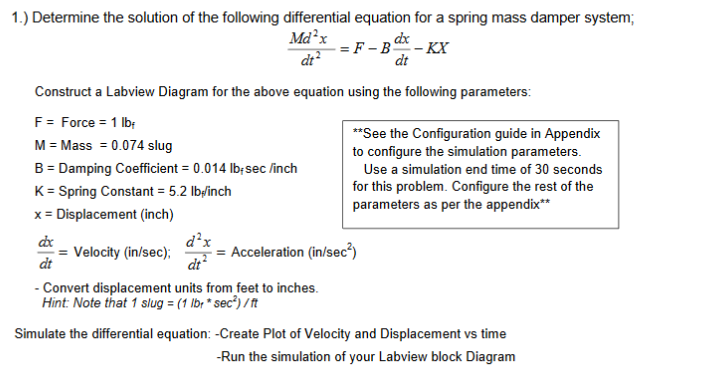
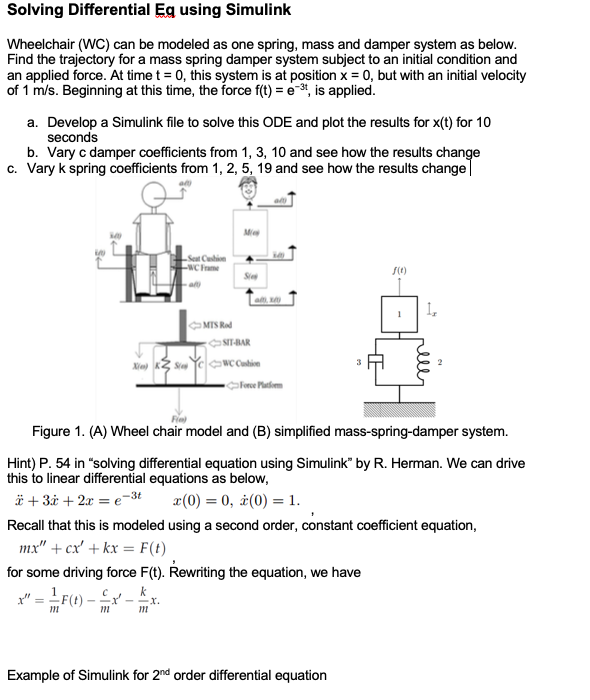
Function dydt odefun x F 1x 13 - 1x 12 1x 1. K 361 107. What is the general solution to the differential equation describing a mass-spring-damper. F t l y dt dy R dt d y M λ 2 2. BACKGROUND MATH The time domain equation of motion for the mass-spring-damper is represented by Newtons Second Law written as the fol-lowing force balance on a structure 𝐌 𝐱 𝐂 𝐱 𝐊 𝐱 M Mass C Damping K Stiffness. Presents a canonical mass-spring-damper system and derives the governing differential equation. From the above equation it is clear that the period of oscillation is free from both gravitational acceleration and amplitude. One can buy dampers the shock absorbers in your car contain dampers. A spring-mass-damper-system is an approximation of a dynamic system having an energy conserving spring part an energy dissipating damper and an inertial mass part.

K 361 107. Similar to FEM the whole body is discretised into springs and dampers in series or parallel with. The damping coefficient c is simply defined as the damping force divided by shaft velocity. Because the roots are repeated the system is critically damped. Based on Newtons second law. Divide the equation through by m. Ylabel Position Speed m ms xlabel Time s title mass-spring-damper system.